The UPSC Mathematics Optional Syllabus is designed for candidates aspiring to take the Civil Services Examination. It encompasses a comprehensive curriculum that covers various mathematical concepts and principles essential for advanced studies. The syllabus includes topics such as Algebra, Real Analysis, Linear Programming, and Statistics, along with an emphasis on the application of mathematical theories to solve complex problems. Candidates are expected to demonstrate proficiency in both theoretical knowledge and practical problem-solving skills. This UPSC Maths Optional Syllabus not only prepares students for the examination but also enhances their analytical and logical reasoning capabilities, crucial for effective decision-making in administrative roles.
UPSC Maths Optional Syllabus 2025
detailed syllabus for the UPSC Maths Optional Syllabus 2025 Civil Services Exam. This syllabus is divided into Paper 1 and Paper 2, each covering a variety of topics.
Paper 1:
- Linear Algebra:
- Vector spaces, linear dependence and independence, subspaces, bases, dimensions.
- Matrix algebra, rank of a matrix, inverse, linear transformations, rank-nullity theorem.
- Eigenvalues and eigenvectors, Cayley-Hamilton theorem.
- Diagonalization, Jordan and canonical forms.
- Calculus:
- Real numbers, limits, continuity, and differentiability.
- Mean value theorems, Taylor’s theorem with remainders.
- Limit, continuity, and differentiability of functions of two or more variables, partial derivatives, maxima, and minima.
- Riemann integration, fundamental theorem of calculus, improper integrals, double and triple integrals (applications included).
- Analytic Geometry:
- Cartesian and polar coordinates in two and three dimensions.
- Second-degree equations in two dimensions, reduction to canonical forms, and classification of conics.
- The equation of a plane, sphere, paraboloid, ellipsoid, and hyperboloid.
- Curves in space, curvature, and torsion, Frenet’s formulae.
- Ordinary Differential Equations:
- Formation of differential equations, order and degree.
- First order differential equations (linear and exact), integrating factors, orthogonal trajectories.
- Second and higher-order linear differential equations with constant coefficients.
- Simultaneous linear differential equations, method of variation of parameters.
- Applications to simple problems in mechanics, electrical circuits, and geometrical optics.
- Dynamics & Statics:
- Rectilinear and planar motion, Newton’s laws, conservation of energy, momentum, and work-energy theorem.
- Motion under central forces, Kepler’s laws.
- Equilibrium of a system of particles, work and potential energy.
- Virtual work, Lagrange’s equations for holonomic systems.
- Vector Analysis:
- Scalar and vector fields, gradient, divergence, and curl.
- Line and surface integrals, Green’s, Stokes’, and Gauss’ theorems.
Paper 2:
- Algebra:
- Groups, subgroups, Lagrange’s theorem, homomorphism, and isomorphism.
- Permutations, cyclic groups, Cayley’s theorem.
- Rings, ideals, integral domains, fields, quotient rings.
- Field extensions, Galois theory.
- Real Analysis:
- Real number system as a complete ordered field, sequences and series.
- Continuity, uniform continuity, properties of continuous functions on closed intervals.
- Riemann-Stieltjes integration, uniform convergence.
- Differentiation of functions, mean value theorem, convergence of sequences and series of functions.
- Functions of several variables: continuity, differentiation, multiple integrals.
- Complex Analysis:
- Analytic functions, Cauchy-Riemann equations.
- Cauchy’s theorem, Cauchy’s integral formula.
- Taylor and Laurent series, residues, contour integration.
- Linear Programming:
- Formulation of linear programming, graphical and simplex methods.
- Duality, transportation and assignment problems.
- Partial Differential Equations:
- Formation and classification of partial differential equations.
- First-order partial differential equations and their solutions.
- Second-order linear equations and classification.
- Method of separation of variables, application to heat, wave, and Laplace equations.
- Numerical Analysis & Computer Programming:
- Numerical methods: solutions of algebraic and transcendental equations.
- Interpolation, numerical differentiation and integration, solution of linear systems.
- Basics of computer programming (simple algorithms and flowcharts).
- Mechanics and Fluid Dynamics:
- Generalized coordinates, Lagrange’s equations.
- Hamilton’s equations, moment of inertia, the motion of rigid bodies.
- Equations of continuity, Euler’s equations of motion for inviscid flow, Bernoulli’s equation.
- Statistics & Probability:
- Probability theory, conditional probability, Bayes’ theorem.
- Discrete and continuous random variables, moments, and distributions.
- The law of large numbers, central limit theorem.
- Sampling distributions, estimation, hypothesis testing.
The UPSC Mathematics Optional syllabus requires a comprehensive understanding of both theoretical and applied mathematics, making it essential to practice problem-solving for the exam.
UPSC Mathematics Syllabus for Optional Paper 1 in Details
UPSC CSE Maths Optional Paper 1 carries a weightage of 250 marks. The UPSC Maths syllabus is organized in the following order: Linear Algebra, Calculus, and Analytic Geometry (Section A), Ordinary Differential Equations, Vector Analysis and Dynamics, and Statics (Section B).
| SECTION A | |
| Linear Algebra | Vector spaces over R and C, linear dependence and independence, subspaces, bases, dimensions, Linear transformations, rank and nullity, matrix of a linear transformation. Algebra of Matrices; Row and column reduction, Echelon form, congruence’s and similarity; Rank of a matrix; Inverse of a matrix; Solution of system of linear equations; Eigenvalues and eigenvectors, characteristic polynomial, Cayley-Hamilton theorem, Symmetric, skew-symmetric, Hermitian, skew-Hermitian, orthogonal and unitary matrices and their eigenvalues. |
| Calculus | Real numbers, functions of a real variable, limits, continuity, differentiability, mean-value theorem, Taylor’s theorem with remainders, indeterminate forms, maxima and minima, asymptotes; Curve tracing; Functions of two or three variables; Limits, continuity, partial derivatives, maxima and minima, Lagrange’s method of multipliers, Jacobian. Riemann’s definition of definite integrals; Indefinite integrals; Infinite and improper integral; Double and triple integrals (evaluation techniques only); Areas, surface and volumes. |
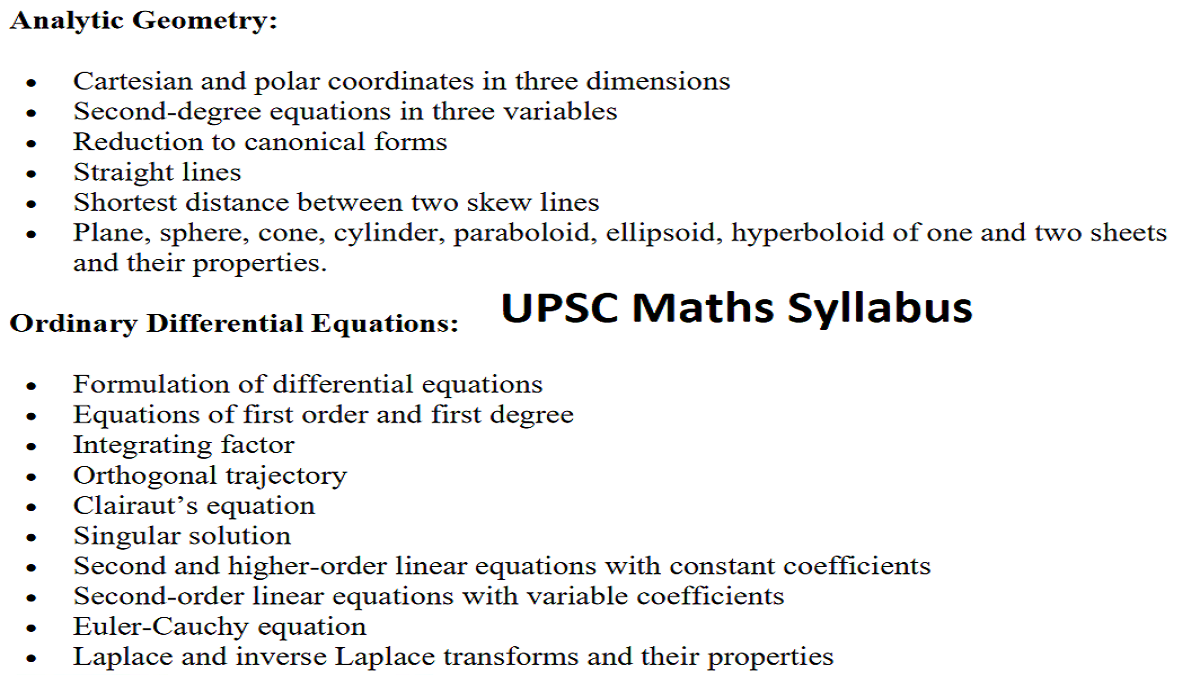
| Analytic Geometry | Cartesian and polar coordinates in three dimensions, second degree equations in three variables, reduction to Canonical forms; straight lines, shortest distance between two skew lines, Plane, sphere, cone, cylinder, paraboloid, ellipsoid, hyperboloid of one and two sheets and their properties. |
| SECTION B | |
| Ordinary Differential Equations | Formulation of differential equations; Equations of first order and first degree, integrating factor; Orthogonal trajectory; Equations of first order but not of first degree, Clairaut’s equation, singular solution. Second and higher order linear equations with constant coefficients, complementary function, particular integral and general solution. Second order linear equations with variable coefficients, Euler-Cauchy equation; Determination of complete solution when one solution is known using method of variation of parameters. Laplace and Inverse Laplace transforms and their properties, Laplace transforms of elementary functions. Application to initial value problems for 2nd order linear equations with constant coefficients. |
| Vector Analysis | Scalar and vector fields, differentiation of vector field of a scalar variable; Gradient, divergence and curl in cartesian and cylindrical coordinates; Higher order derivatives; Vector identities and vector equation. Application to geometry: Curves in space, curvature and torsion; Serret-Frenet’s formulae. Green’s, Gauss and Stokes’ theorems. |
| Dynamics and Statics | Rectilinear motion, simple harmonic motion, motion in a plane, projectiles; Constrained motion; Work and energy, conservation of energy; Kepler’s laws, orbits under central forces. Equilibrium of a system of particles; Work and potential energy, friction, Common catenary; Principle of virtual work; Stability of equilibrium, equilibrium of forces in three dimensions. |
UPSC Maths Optional Syllabus 2024 for Paper 2 in details
Maths Optional Paper-2, weightage 250 marks. Modern Algebra, Real Analysis, Complex Analysis, and Linear Programming (Section A) should come first, then Partial Differential Equations, Numerical Analysis and Computer Programming, and Mechanics and Fluid Dynamics.
| SECTION A | |
| Modern Algebra | Groups, subgroups, cyclic groups, cosets, Lagrange’s Theorem, normal subgroups, quotient groups, homomorphism of groups, basic isomorphism theorems, permutation groups, Cayley’s theorem. Rings, subrings and ideals, homomorphisms of rings; Integral domains, principal ideal domains, Euclidean domains and unique factorization domains; Fields, quotient fields. |
| Real Analysis | Real number system as an ordered field with least upper bound property; Sequences, limit of a sequence, Cauchy sequence, completeness of real line; Series and its convergence, absolute and conditional convergence of series of real and complex terms, rearrangement of series. Continuity and uniform continuity of functions, properties of continuous functions on compact sets. Riemann integral, improper integrals; Fundamental theorems of integral calculus. Uniform convergence, continuity, differentiability and integrability for sequences and series of functions; Partial derivatives of functions of several (two or three) variables, maxima and minima. |
| Complex Analysis | Analytic function, Cauchy-Riemann equations, Cauchy’s theorem, Cauchy’s integral formula, power series, representation of an analytic function, Taylor’s series; Singularities; Laurent’s series; Cauchy’s residue theorem; Contour integration. |
| Linear Programming | Linear programming problems, basic solution, basic feasible solution and optimal solution; Graphical method and simplex method of solutions; Duality. Transportation and assignment problems. |
| SECTION B | |
| Partial Differential Equations | Family of surfaces in three dimensions and formulation of partial differential equations; Solution of quasilinear partial differential equations of the first order, Cauchy’s method of characteristics; Linear partial differential equations of the second order with constant coefficients, canonical form; Equation of a vibrating string, heat equation, Laplace equation and their solutions. |
| Numerical Analysis
And Computer Programming |
Numerical methods: solution of algebraic and transcendental equations of one variable by bisection, Regula-Falsi and Newton-Raphson methods; solution of system of linear equations by Gaussian elimination and Gauss-Jordan (direct), Gauss-Seidel(iterative) methods. Newton’s (forward and backward) interpolation, Lagrange’s interpolation. Numerical integration: Trapezoidal rule, Simpson’s rules, Gaussian quadrature formula. Numerical solution of ordinary differential equations: Euler and Runge Kutta-methods. Computer Programming: Binary system; Arithmetic and logical operations on numbers; Octal and Hexadecimal systems; Conversion to and from decimal systems; Algebra of binary numbers. Elements of computer systems and concept of memory; Basic logic gates and truth tables, Boolean algebra, normal forms. Representation of unsigned integers, signed integers and reals, double precision reals and long integers. Algorithms and flow charts for solving numerical analysis problems. |
| Mechanics and Fluid Dynamics | Generalised coordinates; D’Alembert’s principle and Lagrange’s equations; Hamilton equations; Moment of inertia; Motion of rigid bodies in two dimensions. Equation of continuity; Euler’s equation of motion for inviscid flow; Stream-lines, path of a particle; Potential flow; Two-dimensional and axisymmetric motion; Sources and sinks, vortex motion; Navier-Stokes equation for a viscous fluid. |
UPSC Maths Syllabus 2025 PDF Download
The UPSC Mathematics syllabus for the 2025 Civil Services Examination consists of two papers, each carrying a weightage of 250 marks. The syllabus is well-defined and covers a variety of topics essential for candidates opting for Mathematics as their optional subject. Download UPSC Maths Syllabus 2025 PDF Link soon.
Benefits of Choosing Maths as an Optional Subject in UPSC Mains Exams
Choosing Mathematics as an optional subject for the UPSC Civil Services Mains Exam offers several advantages:
Objectivity and Consistency in Scoring
- Mathematics is a highly objective subject. Unlike humanities subjects where marks can vary due to interpretation, maths answers are based on clear calculations. This allows for consistent scoring, as there is often only one correct answer.
No Subjective Evaluation
- In Mathematics, either the solution is correct, or it is not, leaving little room for subjective marking. This minimizes variations in scores and reduces bias, which can be common in other optional subjects.
Overlapping Topics with Other Exams
- Mathematics optional helps candidates preparing for exams like the Indian Statistical Service, Indian Economic Service, and other competitive exams that include quantitative aptitude. This synergy allows candidates to streamline their preparation for multiple exams.
Availability of Study Resources
- The syllabus for Mathematics in the UPSC Mains aligns with standard undergraduate (B.Sc.) level books. Since these resources are widely available and standardized, candidates can rely on well-defined study material.
Less Competition and Fewer Takers
- Fewer candidates opt for Mathematics compared to popular subjects like Geography or Public Administration, which may increase the chances of standing out if the candidate scores well.
High Scoring Potential for Well-Prepared Candidates
- With the right preparation, Mathematics can be very scoring. Candidates who are strong in concepts and calculations can achieve high marks, which significantly contributes to the overall score in the UPSC Mains.
Develops Analytical and Problem-Solving Skills
- Preparing for Mathematics enhances analytical thinking and problem-solving skills, which are useful in both the Prelims and the Mains (especially for the CSAT paper) and for handling complex problems during service.
Predictable Syllabus and Questions
- The syllabus for Mathematics has a fixed scope, and question patterns are often similar year-to-year. This predictability allows candidates to prepare thoroughly with practice on past papers and focus on specific question types.
Supports Logical Approach in GS and Essay Papers
- Mathematics encourages logical and structured thinking, which is beneficial for other parts of the UPSC exam, like General Studies and the Essay paper, where clear structuring of ideas and arguments is necessary.
While Maths has many benefits, it is also demanding and requires a clear understanding of concepts and consistent practice. Candidates should choose it only if they have a strong foundation and genuine interest in the subject.
UPSC Maths Optional Syllabus Preparation Strategy
The UPSC Maths Optional syllabus can be demanding but rewarding, as it offers a clear, objective scoring potential for those with a strong mathematical background. Here’s a structured strategy to tackle the syllabus effectively:
Understand the Syllabus Thoroughly
- The UPSC Maths Optional syllabus is divided into two papers:
- Paper 1: Linear Algebra, Calculus, Analytic Geometry, Ordinary Differential Equations, Dynamics & Statics, and Vector Analysis.
- Paper 2: Algebra, Real Analysis, Complex Analysis, Linear Programming, Partial Differential Equations, and Numerical Analysis & Computer Programming.
- Go through each topic in the syllabus carefully and understand the weightage of each section. Some areas are vast and may require more time, like Calculus and Algebra, while others, such as Vector Analysis, are relatively smaller.
Gather Quality Resources
- Books: Select a few standard textbooks for each topic. Some recommended books include:
- Linear Algebra: Hoffman and Kunze, Schaum’s Outline for practice.
- Calculus: Gorakh Prasad for calculus, Thomas and Finney for additional problems.
- Real Analysis: Malik and Arora, Shanti Narayan.
- Complex Analysis: Churchill and Brown.
- Ordinary Differential Equations: Raisinghania.
- Previous Year Question Papers: Analyze past UPSC papers to understand the pattern, frequently asked questions, and the difficulty level.
Make a Study Plan
- Weekly Goals: Divide the syllabus into small, manageable portions and set weekly goals. Aim to cover each topic thoroughly while leaving time for revision.
- Daily Routine: Dedicate 3-4 hours each day for Maths Optional preparation, covering theory, problem-solving, and revisions.
- Time Management: Prioritize topics that are frequently asked in the exam. Ensure enough time for the heavier topics while not neglecting the smaller sections.
Focus on Conceptual Clarity
- Mathematics requires a solid understanding of concepts rather than rote memorization. For each topic:
- Understand the fundamental theories and principles.
- Solve problems at varying difficulty levels to ensure conceptual clarity.
- Revise regularly to reinforce core concepts.
Practice Answer Writing
- Time-bound Practice: Practicing under timed conditions helps improve speed and accuracy, both crucial in the exam.
- Steps in Solutions: UPSC examiners appreciate well-structured answers, so practice writing clear, step-by-step solutions.
- Use Diagrams: For topics like Analytic Geometry, Visual Representation is essential. Practice sketching accurate diagrams where applicable.
Revise Regularly
- Schedule weekly and monthly revision sessions. Use summary notes and formula sheets for quick recaps.
- Solve previous year question papers every few weeks as part of the revision to gauge your preparation level.
Take Mock Tests
- After covering 50-70% of the syllabus, start taking full-length mock tests.
- Analyze your mistakes after each test, focusing on weak areas in your revisions.
Stay Updated with Strategies and Forums
- Join study groups or online forums where you can discuss difficult questions or new strategies. This will help you stay motivated and informed about common mistakes and effective approaches.
Suggested Timeline for Preparation:
- Month 1-3: Cover Paper 1 topics, including Linear Algebra, Calculus, and Vector Analysis.
- Month 4-6: Cover Paper 2 topics, focusing on Real Analysis, Complex Analysis, and Algebra.
- Month 7: Focus on solving previous years’ questions and revising thoroughly.
- Month 8-9: Take mock tests, revise, and fine-tune your time management skills.
By maintaining consistency, emphasizing problem-solving skills, and regularly revising, you’ll be well-prepared to tackle the Maths Optional papers in UPSC.
Books to Study for UPSC Mathematics Optional Syllabus 2025
For the UPSC Mathematics Optional, focusing on the right resources is crucial due to the depth and complexity of the syllabus. Here is a list of recommended books covering key topics:
Linear Algebra
- Linear Algebra by Seymour Lipschutz (Schaum’s Outline Series) – This book provides a comprehensive yet straightforward introduction to linear algebra.
- Linear Algebra by K.C. Prasad – Useful for more in-depth concepts and additional problems.
Calculus
- Differential Calculus by Shanti Narayan and P.K. Mittal – Good for fundamental concepts and problem-solving practice.
- Integral Calculus by A.R. Vasishtha – This covers essential integrals and definite integrals.
Analytic Geometry
- Coordinate Geometry by S.L. Loney – Covers straight lines, circles, and conics in two dimensions in detail.
- Analytic Geometry by N. Saran and R.S. Gupta – Provides a clear approach to 3D geometry topics.
Ordinary Differential Equations (ODEs)
- Differential Equations by M.D. Raisinghania – A well-structured book for both theory and practical problems in differential equations.
Dynamics and Statics
- Statics by S.L. Loney – Standard reference for statics topics, covering forces, equilibrium, and centers of gravity.
- Dynamics by S.L. Loney – Explains the fundamentals of dynamics, including motion under gravity, projectile motion, and simple harmonic motion.
Vector Analysis
- Vector Analysis and an Introduction to Tensor Analysis by Murray Spiegel – Good for understanding vector calculus and applications.
Algebra
- Abstract Algebra by I.N. Herstein – Covers groups, rings, and fields with clarity.
- Higher Algebra by H.S. Hall and S.R. Knight – Useful for complex number theory, quadratic equations, and determinants.
Real Analysis
- Mathematical Analysis by S.C. Malik and Savita Arora – Covers sequences, series, and continuity in a structured way.
- Principles of Mathematical Analysis by Walter Rudin – Often recommended for a deeper understanding, though it’s quite advanced.
Complex Analysis
- Theory of Functions of a Complex Variable by Shanti Narayan – Concise and covers topics relevant to the syllabus.
- Complex Analysis by L.V. Ahlfors – Ideal for advanced learners, though it may be more detailed than required.
Linear Programming and Optimization
- Operations Research by Kanti Swarup, P.K. Gupta, and Man Mohan – Covers linear programming, the simplex method, and other optimization techniques.
Mechanics and Fluid Dynamics
- A Textbook of Fluid Mechanics and Hydraulic Machines by R.K. Bansal – Covers the basics of fluid dynamics.
- Mechanics by P.D. Sharma – Provides good coverage of classical mechanics required for the syllabus.
Additional Preparation Tips:
- Practice Previous Year Papers: Solving previous UPSC question papers is invaluable for understanding the exam’s demands.
- Revision Notes: Make concise notes of formulas, theorems, and important derivations.
- Online Resources and Lectures: Supplementary online resources or lectures may also clarify difficult concepts.
Each of these books has been selected to provide the depth and breadth needed for the UPSC Mathematics Optional. Happy studying, and best of luck!
FAQs
- What are the key topics covered in the UPSC Mathematics Optional Syllabus?
- The syllabus includes areas such as Algebra, Real Analysis, Calculus, Linear Programming, Statistics, and Mechanics. Specifically, topics like complex numbers, matrices, calculus of several variables, differential equations, and probability distributions are essential.
- How is the Mathematics Optional paper structured in the UPSC exam?
- The Mathematics Optional consists of two papers, each carrying 250 marks. Paper I typically focuses on topics like Algebra and Real Analysis, while Paper II emphasizes Calculus, Statistics, and other applied mathematics areas.
- What are some recommended books for preparing for the UPSC Mathematics Optional?
- Recommended texts include “Higher Algebra” by Hall and Knight, “Calculus” by Tom M. Apostol, “Introduction to Real Analysis” by Bartle and Sherbert, and “Mathematical Statistics” by J. Rao. Additionally, NCERT books and past UPSC papers can be beneficial.
- Is it necessary to have a strong background in mathematics to choose it as an optional subject?
- While having a solid foundation in mathematics helps, it is not strictly necessary. Candidates should be comfortable with mathematical concepts and problem-solving. Dedication to consistent practice and understanding of the syllabus is key.
- How can I effectively manage my time while preparing for the Mathematics Optional subject?
- Effective time management involves creating a study schedule that allocates time for each topic based on its complexity and your familiarity with it. Regular practice through solving previous years’ question papers and taking mock tests can also help enhance time management skills during preparation and the actual exam.